CHI-SQUARE

This frequently used test can tell us whether there is a difference in the proportion of a categorical variable that deviates from what would be expected if the variable were evenly distributed among the different groups, as the null hypothesis would state. It goes by the “goodness-of-fit” test because it assesses whether or not the observed data fit an expected pattern if the null hypothesis is correct. It also is used to check for trends.

The formula for the chi-square statistic χ² is a sum of the differences in each cell from what is observed versus what would be expected if the null hypothesis were true. It can be extremely complicated as the number of cells increases. The statistic may be large—into the double or even triple digits. The larger the statistic, the less likely that the null is true and that you would get these results in a random sample. Figure 12-1 is an example of a simple dataset which is used to answer the question: “Is there a significant difference between males and females with respect to their likelihood of being in poverty?”

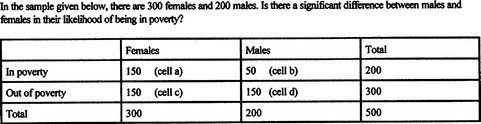
FIGURE 12-1 If there were no difference between males and females with respect to their likelihood of being poor, the proportion of males and females in poverty should not be significantly different. See www.brynmawr.edu/Acads/GSSW/Vartanian/Handouts/ChiSqu.Examples 2007 for full explanation.

Only the one-tail test can be done with the chi-square statistic. Also, if there are more than two groups and we get a statistically significant result, we can only say that as least one of the groups is significantly different from the rest. To make comparisons among all the groups requires additional statistical methods.

THE STUDENT’S T-TEST

This test can be used to see whether there is a difference between sample means in two groups if the variables are continuous. It is used to test for a significant difference due to treatment, or to see whether there has been a change in one group before and after treatment.

The sample is divided into two groups—one that gets an intervention, for example, and one that does not. If the intervention has no effect, then the means of the outcome variable in the two groups would not differ appreciably. When one sample mean is subtracted from the other, the difference is close to zero. The null hypothesis states that the means of the outcome variables in the two groups will be the same and that the treatment difference is zero.

The t statistic is analogous to the Z statistic and, as the sample gets larger, the distribution for t resembles the Z distribution. We can perform a two-tailed test with these distributions, so we can test for a difference in either direction.

CORRELATION

In the real world, we observe that certain variables seem to be connected or related. For instance, hair color and eye color tend to be linked. Variables that are linked together are said to correlate

Stay updated, free articles. Join our Telegram channel

Join