and Vladimir V. Apanasovich1
(1)
Department of Systems Analysis and Computer Simulation, Belarusian State University, Minsk, Belarus
Abstract
In modern fluorescence fluctuation spectroscopy, the autocorrelation function and photon counting distribution are two widely used statistical characteristics of the measured fluctuating fluorescence intensity signal. Applying special analysis methods such as fluorescence correlation spectroscopy (FCS) and photon counting histogram (PCH) to these properties, it is possible to recover values of different parameters of fluorescent molecules such as the concentration, diffusion coefficient, molecular brightness, and kinetic rate constants. The development of new analysis methods is senseless without testing their validity, accuracy, and robustness. The most appropriate check of a method is its application to experimental data. However, sometimes it is more convenient and easier to verify a method on simulated data. Simulation is also useful for better understanding the processes that were modeled during the development of analysis methods. Here, we present two simulation models providing an autocorrelation function and photon counting distribution of a sequence of photon arrival times detected in fluorescence fluctuation spectroscopy.
Key words
Fluorescence fluctuation spectroscopyFluorescence correlation spectroscopyPhoton counting histogramAutocorrelation functionSimulationStochastic point process1 Introduction
Fluorescence fluctuation spectroscopy (FFS) methods are widely used in modern biophysical and biochemical research [1]. In FFS the information about dynamics and interactions of fluorescently labeled macromolecules is extracted from the detected fluorescence intensity fluctuations. These fluctuations are caused by various kinetic processes, which alter the number of molecules in a small observation volume and its intrinsic fluorescence properties. A detected fluorescence signal thus contains information about molecular diffusion, photophysical, and chemical dynamics [2–5]. In FFS the commonly used methods for extracting this information are fluorescence correlation spectroscopy (FCS) [6], photon counting histogram (PCH) analysis [7, 9], fluorescence intensity distribution analysis (FIDA) [8] and PCH with out-of-focus correction [9]. FCS extracts the information about the diffusion coefficients, chemical kinetics, and excited-state dynamics of fluorescent molecules at picomolar concentration from the analysis of a temporal autocorrelation function (ACF) of the measured sequence of photon arrival times. Both PCH and FIDA are used to obtain information on the concentration and specific brightness of fluorescent molecules and are based on the least-squares analysis of photon counting distribution (PCD), calculated from the same measured sequence of photon arrival times.
The development of new data analysis methods in FFS requires comprehensive testing of their validity, accuracy, resolvability, and robustness. The testing can be performed by applying a method to data obtained from real experiments. It is also convenient to perform preliminary testing of an analysis method on simulated data, because the use of these data can assist in interpretation and prediction of real experimental results. One typical application of simulations can be the verification of the resolvability of the method under a given set of parameters and the signal-to-noise ratio (S/N).
There are various simulation models of ACF and PCD taking into account translational and anomalous diffusion of fluorescent molecules, photobleaching, triplet-state dynamics, and non-Gaussian brightness profiles [10–12]. All these models are based on sequential simulation of the number of photon counts within a short time interval (or bin time). Therefore, the output of these models is the already binned intensity trace, which is not suitable for calculating ACF and PCD at arbitrary binning times, since binning times must be equal to or a multiple of this interval.
In this chapter we describe two simulation models. The first one simulates translational diffusion of individual fluorescent molecules and their transitions into a non-radiative or dark state (flickering), which are the main processes in fluctuation spectroscopy, and thus enables to obtain a “complete” stream of photon arrival times in FFS. A distinctive feature of this model is that the sequence of photon arrival times is considered as a doubly stochastic Poissonian point process (DSP) [7]. The advantage of such approach is the ability to calculate ACF and PCD and other statistical characteristic of the point process at an arbitrary binning time [13]. The model is well suitable for parallel simulation of photon arrival times from several types of independent molecules, provided that they will be combined into one common point process afterwards. Parallel simulation enables to simulate a point process with long duration and high concentration of molecules. It should be noted that the model is not valid at very high concentrations when the distance between fluorescent molecules becomes shorter and their intermolecular interaction becomes sufficient. The second model was specially developed for the fast simulation of PCD. It enables to simulate the already binned intensity trace from which only the photon counting distribution with given parameters can be calculated. This model does not simulate the diffusion of individual molecules and therefore is simpler and much faster than the first one. The calculation of ACF from the simulated intensity trace is still possible but unreasonable because the information about the diffusion is not involved in the simulation. The model is based on the idea that the number of molecules in the open observation volume and the number of photons emitted by a single molecule follow the Poisson distribution. In addition, it enables to simulate the signal from the “out-of-focus” molecules that opens the possibility to test the analysis methods with the out-of-focus correction [9].
2 Methods
2.1 Simulation of an Autocorrelation Function and Photon Counting Distribution via a Doubly Stochastic Poissonian Point Process
Here we describe the method of simulation of ACF and PCD via DSP. Free translational diffusion of fluorescent molecules through the inhomogeneous Gaussian-shaped observation volume and their transitions into a non-radiative state (flickering) are simulated. Initial model parameters are the dimensions of the modeling area L x , L y , L z in μm (L x > ω 0, L y > ω 0, L z > z 0), rate constants k AB, k BA of transitions of molecules into non-radiative state A → B and back B → A in s−1, molecular brightness q in counts per second per molecule (cpsm), diffusion coefficient D in m2/s, concentration С in nmol/L (nM), and the start and the end modeling time T 0 and T m in seconds. The constants ω 0 and z 0 (in μm) are parameters of the three-dimensional Gaussian brightness profile [14]
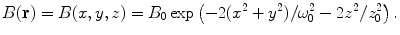
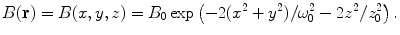
(1)
The description of the general scheme of simulation is given below:
1.
Set the dimensions of the modeling region and the parameters of the brightness profile B(r).
2.
Increase the molecule brightness according to further losses due to transitions of molecules into the non-radiative state q s = (1 + P B)q, where P B = k AB/(k AB + k BA). Here q is the time-independent “true” brightness (B 0 = 1 because no brightness profile correction is applied; see Chapter 33 by Skakun et al. in the same volume for details).
3.
Get the number of molecules N in the modeling area. The total number of molecules is calculated as the integer closest to the value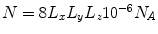 
if the concentration is given in nmol/L, where N A = 6.022 × 1023 mol−1 is Avogadro’s constant.
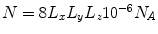if the concentration is given in nmol/L, where N A = 6.022 × 1023 mol−1 is Avogadro’s constant.4.
Obtain a sequence of photon arrival times (further simply denoted as events) from a single diffusing molecule switching between radiative and non-radiative states. It is modeled as DSP. One way to simulate such a process is to reject the events of the stationary Poissonian point process of higher intensity according to the processes that form the second stochastic:
(a)
Set the initial coordinates of the molecule according to uniform distribution in the modeling region (see Note 1 for details).
(b)
Set the initial state of the molecule (radiative A or non-radiative B) by generation of the random value α uniformly distributed on the interval (0,1) (further simply a basic random value) and checking the condition α ≤ P A, where P A = k BA/(k AB + k BA). If the condition is true, then the state is radiative A, else, non-radiative B (see Note 2 for details).
(c)
Simulate the event of the stationary Poissonian point process on the time interval [T 0; T m] with the intensity λ ≥ q s. The event t j of the Poissonian point process can be calculated using the recurrent formula t j = t j − 1 − λ −1 ln α, where t 0 = T 0 and α is the basic random value.
(d)
Simulate molecular diffusion within the time step Δt = t j − t j − 1. The molecule coordinates x, y, z are shifted with increments Δx, Δy, Δz that are Gaussian-distributed values with mean μ = 0 and variance σ 2 = 2DΔt [15].
(e)
Check the border conditions. One widespread kind of the border conditions is the periodic condition: if a molecule leaves the modeling region, it returns from its opposite side (see Note 3 for details).
(f)
Check the condition β j ≤ Ψ(t j ), where β j is a random value, uniformly distributed on [0; λ], and Ψ(t j ) = q s B(r) is the intensity of photon emission at the point with radius vector r at the time t j . If this condition is true, go to step g; else, ignore the event t j and go to step c.
(g)
Check the radiative state of the molecule at time t j . If the molecule is in the B state, then the event t j is ignored. This can be done by the generation of an alternate sequence of “on/off” intervals during which the molecule is in either A or B state. This sequence is continuously generated starting from the initial time T 0. The length of the “on” interval is modeled as Δt A = −τ Aln α, and the length of the “off” interval is correspondently modeled as Δt B = −τ Bln α, where α is the basic random value, τ A = 1/k AB, and τ B = 1/k BA (see Note 2).
(h)
The generation of events (steps c–g) is continued while t j < T m. The step c forms the first stochastic of the point process, the so-called shot noise. The next four steps (d–g) are intended for the simulation of physical processes (diffusion of molecules through the illuminated volume and their blinking) that forms the second stochastic of the double-stochastic Poissonian point process.
5.
Repeat step 4 for every molecule.
6.
Combine all obtained sequences into the resulting sequence with events sorted in ascending order.
7.
Calculate ACF, PCD, or other characteristics (e.g., fluorescence factorial cumulants [16]) from the simulated sequence of photon arrival times.
In order to simulate the photon arrival times from a mixture of different molecules, the steps of the algorithm have to be performed independently for each type of molecules. The two last steps have to be done only once in the end of the simulation.
Several simulations have been performed in order to validate the proposed model. The idea was to demonstrate the ability of the model to reproduce real measured data on the basis of the analyzed results. The initial parameters of the model have been set to the values obtained after the global analysis of the solution of monomeric and dimeric forms of eGFP in aqueous buffer solution (see details in Chapter 33 by Skakun et al. in the same volume). From the simulated point processes, ACF and two PCDs were calculated and then analyzed globally (separately for the monomer and dimer) accordingly to the protocol described in Chapter 33 by Skakun et al. in the same volume. The analysis was performed in the FFS data processor (http://www.sstcenter.com/software/index.phtml?pageid=idFFSDPFeatures) [17].
The results of analysis of simulated data are shown in Fig. 1 and in Table 1. Good correspondence in the shape of analyzed data and fit parameter values was obtained for the autocorrelation function. However, the experimental PCDs differ from the simulated ones. It could be expected as the model described here does not simulate the out-of-focus emission that was accounted for in the analysis of the measured data. Therefore, we used the “true” brightness in our simulations in order to get rid of the influence of the correction parameter on the brightness value. The correction parameter was set to zero in the analysis of the simulated data.
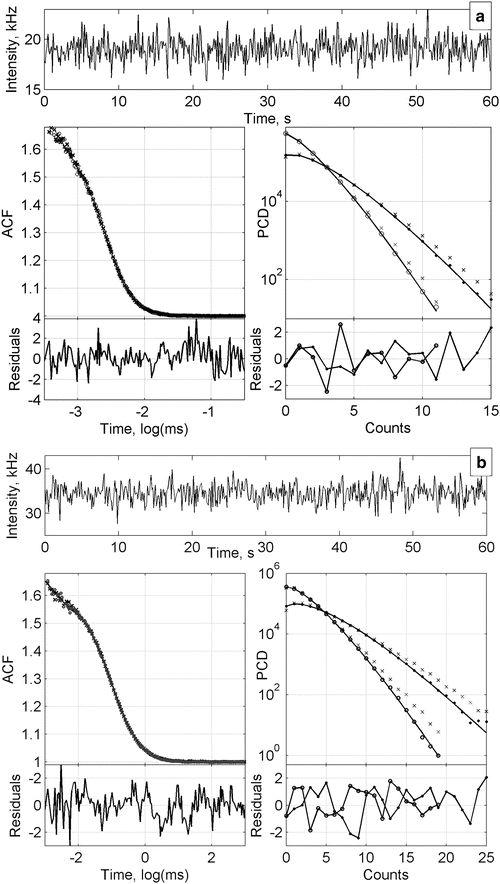
Fig. 1
The results of global analysis of ACF and PCD simulated by the first model. The intensity of the simulated sequence of photon arrival times is plotted on the top of each panel. The residuals are plotted in the bottom and both simulated and theoretical ACF and PCD are plotted in the middle. Simulation was performed with parameters given in Table 1. To reproduce the real experiment, the model parameter values were taken from the analysis of eGFP monomer (a) and dimer (b) described in Chapter 33 by Skakun et al. in the same volume. One AFC and two PCDs (circles) calculated from the simulated point processes with the sampling time 50 μs (open circles) and 100 μs (closed circles), respectively, were analyzed using the FFS data processor as described in Chapter 33 by Skakun et al. in the same volume. Measured AFC and PCDs (crosses) are presented for comparison
Stay updated, free articles. Join our Telegram channel
Full access? Get Clinical Tree
