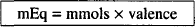Let us go through a couple of problems as a review.

The chemical equation for the dissociation of calcium nitrate (Ca (NO₃)₂) is:

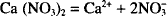
Thus, one mol of calcium nitrate yields 1 mol of calcium ion and 2 mols of nitrate ion. How many mols result from the dissociation of 1 mol of sodium sulfate? (Na₂SO₄)

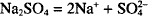
Each mol of sodium sulfate yields 2 mols of sodium and 1 mol of sulfate.

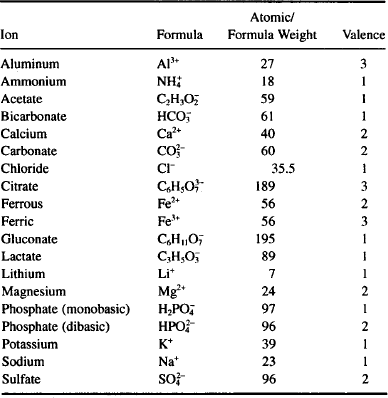
Now it’s your turn to practice, using elementary inorganic chemistry skills and data provided in Table 9.1 and Appendix 4 (names, symbols, atomic weight of some elements with pharmaceutical importance).

TABLE 9.1 Properties of some important ions

How many mols of magnesium chloride (MgCl₂) are necessary to yield 1 mol of magnesium ion?

Solution. MgCl₂ = Mg²⁺+ 2Cl⁻

Each mol of magnesium chloride yields 1 mol of magnesium ion.

Solution. One-half mol of MgCl₂

MgCl₂ = Mg²⁺ + 2Cl⁻

Each mol of magnesium chloride yields 2 mols of chloride ion.

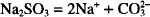
Each mol of sodium carbonate contains 2 mols of sodium. The molecular weight of sodium carbonate is 106. Therefore, 106 g of sodium carbonate contain 46.0 g of sodium.

How many grams of anhydrous sodium carbonate contain 350 mg of sodium?

Solution. 0.807 g

CALCULATIONS

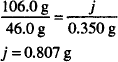
Solution. 40%

Calcium has an atomic weight of 40.0.

CaCO₃ has a molecular weight of 100.0.

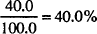
MILLIMOL (MMOL)

If you feel you need a review, check these examples and try the next two problems. Otherwise, go to frame 8.

How many milligrams of sodium chloride represent 0.5 mmol?

Molecular wt of NaCl = 58.5

58.5 mg NaCl = 1 mmol

0.5 mmol = 29.3 mg

How many millimols are there in 3.2 g of calcium fluoride?

MW of CaF₂ = 78.0

78.0 mg CaF₂ = 1 mmol

3200 mg × 1 mmol/78.0 mg = 41 mmol

A prescription calls for 24 mmol of potassium chloride. How many grams of KCl are required?

Solution. 1.79 g

CALCULATIONS

MW of KCl = 74.5 and 1788 mg = 1.79 g

74.6 mg = 1 mmol

24 mmol 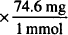= 1790 mg = 1.79 g

7. How many grams of sodium chloride should be used to prepare this solution?

	NaCl solution 90.0 mL
	Each 5 mL should contain 0.6 mmol of Na+.

Solution. 0.632 g

CALCULATIONS

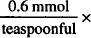18 teaspoonfuls = 10.8 mmol Na⁺

NaCl = Na⁺ + Cl⁻

From this equation we see that each mol of sodium chloride yields 1 mol of Na⁺.

Therefore, 10.8 mmol of Na⁺ will be supplied by 10.8 mmol of NaCl. Thus,

MW of NaCl = 58.5

58.5 mg NaCl = 1 mmol

10.8 mmol 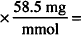632 mg = 0.632 g

EQUIVALENT AND MILLIEQUIVALENT (mEq)

Since total positive and negative charges in a salt must balance, we can define the valence of a salt as the sum of either the positive or negative charges. For example, the valence of CaCl₂, which contains two positive charges and two negative charges, is 2.

Write the valence of each of the following compounds:

Solutions.

Solution. 2 equivalents of each ion.

K₂CO₃ = 2K⁺ + CO₃²⁻

Thus 2 mol of K⁺ are produced

mols × valence = equivalents

2 × 1 = 2 equivalents of K⁺

Only 1 mol of carbonate is produced.

mols × valence = equivalents

1 × 2 = 2 equivalents of CO₃²⁻

Notice that the equivalents of cation and anion are equal in number.

(9.1)

Since the number of millimols of a drug in solution may be calculated by dividing the amount of drug, in mg, by its molecular weight, then the following may also be used:

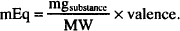
In addition, if we rearrange its terms, the number of milligrams of drug can be determined if a given number of milliequivalents of drug are known:

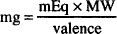
Now, check the following example solved by each approach.

How many mg/mL are present in a solution containing 2 mEq/mL of KCl? (MW = 74.5)

Solving by (a):

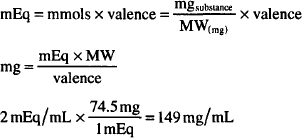
or

Solving by (b):

MW of KCl = 74.5 g

Equiv. weight of KCl = 74.5/1 = 74.5 g (valence = 1)

1 mEq of KCl = 1/1000 × 74.5 g = 0.0745 g = 74.5 mg

2 mEq = 2 × 74.5 mg = 149 mg/mL

Solving by (c):

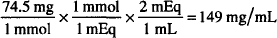
Solution. 16.7 mEq

CALCULATIONS

MW of MgSO₄ = 120

EqW = 120/2 = 60 g

1 mEq = 60 mg

1 g = 1000 mg

1000 mg × 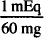= 16.7 mEq

or

MW of MgSO₄ = 120

1 mmol MgSO₄ = 120 mg

EqW = 120 g/2 = 60 g

1 mEq = 1/1000 × 60 g = 0.060 g = 60 mg

1 mmol = 2 mEq (120 = 2 × 60)

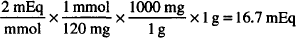
Solution. 3 mg%

CALCULATIONS

Atomic weight of magnesium = 24 g

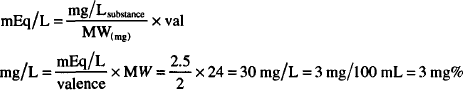
or

Atomic weight of magnesium = 24 g

Eq. weight of Mg²⁺ = 24/2 = 12 g

1 mEq = 12 mg

2.5 mEq/L = 2.5 × 12 = 30 mg/L = 3 mg/100 mL = 3 mg%

Solution. 780 mL

CALCULATIONS

MW of NaCl = 58.5 g

EqW =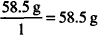

1 mEq = 0.0585 g

2 mEq/kg dose = 2 × 0.0585 = 0.117 g/kg

132 lb = 60 kg (1 kg = 2.2 lb)

0.117 g × 60 = 7.02 g of NaCl needed

0.9% NaCl available = 0.9 g/100 ml

7.02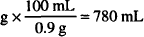

or

132 lb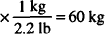

60 kg × 2 mEq/kg = 120 mEq needed

mg =

Solution. 6.02 mEq

CALCULATIONS

KF = K⁺ + F⁻

1 mEq F⁻ = 1 mmol F⁻ = 1 mmol KF = 58.1 mg

350 mg KF × 1 mEq F⁻/58.1 mg KF = 6.02 mEq F⁻

or

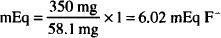
Solution. 2.10 mEq

CALCULATIONS

(5 mL × 0.02 g/mL) = 0.1 g MgCl₂ = 100 mg

MgCl₂ = Mg²⁺ + 2 Cl⁻

1 mmol MgCl₂ = 95.3 mg = 1 mmol Mg²⁺ = 2 mEq Mg²⁺

100 mg MgCl₂ 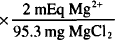= 2.10 mEq Mg²⁺

or

Solution. 6.62 g

CALCULATIONS

2.5 mEq/teaspoonful × 36 teaspoonfuls = 90 mEq needed

CaCl₂ · 2H₂O = Ca²⁺ + 2Cl⁻ + 2H₂O

1 mmol Ca²⁺ = 2 mEq Ca²⁺ = 1 mmol CaCl₂ · 2H₂O = 147.0 mgCaCl₂ · 2H₂O

90 mEq 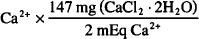= 6620 mg = 6.62 g CaCl₂ · 2 H₂O

17. If you would like more practice, try your hand at these. Check atomic weight, formulas and valences in Table 9.1 and Appendix 4.

A. If 2.86 g of magnesium chloride are used to prepare 120 mL of a solution, how many milliequivalents of magnesium ion will each teaspoon contain?

B. How many grams of potassium chloride should be used to prepare 90 mL of a solution containing 0.8 mEq of K⁺/mL?

C.

	Solution calcium chloride 5%	180 mL
	Sig: 1 teaspoonful tid

How many milliequivalents of chloride ion does the patient receive each day?

D.

	Potassium sulfate to yield 1.0 mEq of K+
	Aqua qs ad	5.0 mL
	d.t.d. #24
	Sig: 5.0 mL b.i.d.

How many grams of potassium sulfate should be used for this prescription?

E. If city water contains 2.5 ppm of NaF, calculate the number of milliequivalents of fluoride ingested by a person who drinks 1.5L of water.

F. A solution is prepared by dissolving 8.42 g of sodium chloride in sufficient water to make 180 mL of solution. In how many milliliters will 8 mEq of sodium ion be contained?

Solutions.

Stay updated, free articles. Join our Telegram channel

Join