Two-Factor Medication Problems
Objectives
After completing this chapter, you will successfully be able to:
Solve two-factor–given quantity to one-factor–wanted quantity medication problems involving a specific amount of medication ordered based on the weight of the patient.
Calculate medication problems requiring reconstitution of medications by using information from a nursing drug reference, label, or package insert.
Solve two-factor–given quantity to two-factor–wanted quantity medication problems involving a specific amount of fluid to be delivered over limited time using an intravenous pump delivering milliliters per hour (mL/hr).
Solve two-factor–given quantity to two-factor–wanted quantity medication problems involving a specific amount of fluid to be delivered over a limited time using different types of intravenous tubing that deliver drops per minute (gtt/min) based on a specific drop factor.
 Preventing Medication Errors
Preventing Medication ErrorsAlthough medications are ordered by physicians or nurse practitioners and administered by nurses using the “six rights of medication administration,” other factors must be considered when administering certain medications or intravenous (IV) fluids.
The weight of the patient often must be factored into a medication problem when determining how much medication can safely be given to an infant or a child or an elderly patient.
The dosage of medication available may be in a powdered form that needs reconstitution to a liquid form before parenteral or IV administration.
Also, the length of time over which medication or IV fluids can be given plays an important role in the safe administration of IV therapy.
To be able to calculate a two-factor–given quantity to one-factor– or two-factor–wanted quantity medication problem, it is important to understand all factors that may need to be considered in some medication problems. With use of dimensional analysis, this chapter will teach you to calculate medication problems involving the weight of the patient, the reconstitution of medications from powder to liquid form, and the amount of time over which medications or IV fluids can be safely administered.
Medication Problems Involving Weight
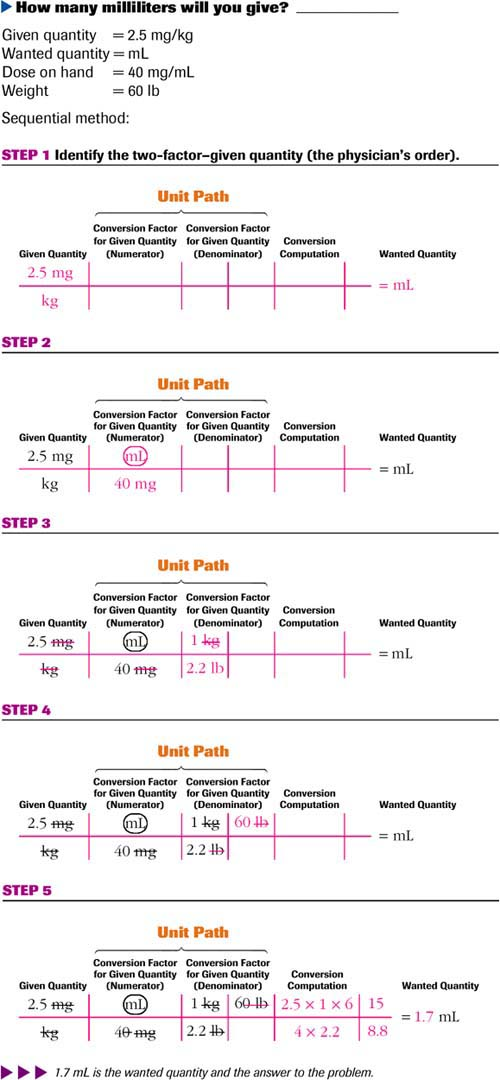
When solving problems with dimensional analysis, you can use either the sequential method or the random method to calculate two-factor–given quantity medication problems. The given quantity (the physician’s order) contains two parts including a numerator (dosage of medication) and a denominator (the weight of the patient). This type of medication problem is called a two-factor medication problem because the given quantity now contains two parts (a numerator and a denominator) instead of just one part (a numerator).
Below is an example of the problem-solving method showing placement of basic terms used in dimensional analysis, applied to a two-factor medication problem involving weight.
 |
 Thinking it Through
Thinking it ThroughThe two-factor–given quantity has been set up with a numerator (2.5 mg) and a denominator (kg) leading across the unit path to a one-factor–wanted quantity with only a numerator (mL).
The dose on hand (40 mg/mL) has been factored in to cancel out the preceding unwanted unit (mg). The wanted unit (mL) is in the numerator and corresponds with the one-factor–wanted quantity (mL).
A conversion factor (1 kg = 2.2 lb) is factored into the unit path to cancel out the preceding unwanted unit (kg).
The weight is finally factored in to cancel out the preceding unwanted unit (lb) in the denominator. All unwanted units are canceled and only the wanted unit (mL) remains and corresponds with the wanted quantity (mL). Multiply the numerators, multiply the denominators, and divide the product of the numerators by the product of the denominators to provide the numerical value.
Example 5.1
The physician orders gentamicin 2.5 mg/kg IV (intravenous) every 8 hours for infection. The vial of medication is labeled 40 mg/mL. The child weighs 60 lb.
 |
 Preventing Medication Errors
Preventing Medication ErrorsOne of the most frequent medication errors is the error made with the conversion of weight.
The weight conversion [1 kg = 2.2 lb] is often incorrectly written [1 lb = 2.2 kg].
Remember that you would rather tell someone your weight in kilograms as it is a much smaller number [1 kg = 2.2 lb or, put in more realistic terms, 90.9 kg = 200 lb].
Dimensional analysis is a problem-solving method that uses critical thinking. When implementing the random method of dimensional analysis, the medication problem can be set up in a number of different ways. The focus is on the correct placement of conversion factors to cancel out all unwanted units. The wanted unit is placed in the numerator to correctly correspond with the wanted quantity.
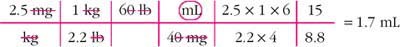 |
Exercise 5.1 Pediatric Medication Problems Involving Weight (See page 137 for answers)
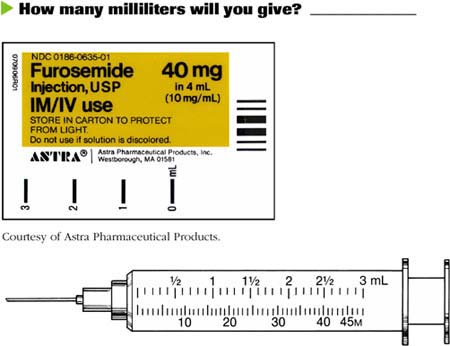
 Order: furosemide 1 mg/kg IV bid for hypercalcemia. The child weighs 45 lb.
Order: furosemide 1 mg/kg IV bid for hypercalcemia. The child weighs 45 lb.
 Order: atropine sulfate 0.01 mg/kg IV stat for bradycardia. The child weighs 20 lb.
Order: atropine sulfate 0.01 mg/kg IV stat for bradycardia. The child weighs 20 lb.

 Order: phenergan 0.5 mg/kg IV every 4 hours prn for nausea. The dose on hand is 25 mg/mL. The child weighs 45 lb.
Order: phenergan 0.5 mg/kg IV every 4 hours prn for nausea. The dose on hand is 25 mg/mL. The child weighs 45 lb.
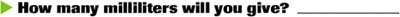
 Order: morphine 50 mcg/kg IV every 4 hours prn for pain. The child weighs 75 lb.
Order: morphine 50 mcg/kg IV every 4 hours prn for pain. The child weighs 75 lb.

 Order: Tagamet 10 mg/kg PO qid for prophylaxis of duodenal ulcer. The dose on hand is 300 mg/5 mL. The child weighs 70 lb.
Order: Tagamet 10 mg/kg PO qid for prophylaxis of duodenal ulcer. The dose on hand is 300 mg/5 mL. The child weighs 70 lb.

Medication Problems Involving Reconstitution
Some medications in vials are in a powder form and need reconstitution before administration. Reconstitution involves adding a specific amount of sterile solution (also called diluent) to the vial to change the powder to a liquid form. Information on how much diluent to add to the vial and what dosage of medication per milliliter will result after reconstitution (also called yield) can be obtained from a nursing drug reference, label, or package insert.
 Preventing Medication Errors
Preventing Medication ErrorsWhen reconstituting medication, always check a nursing drug reference to obtain information regarding the correct diluents and the correct amount of the diluents to be used to prevent medication errors.
Example 5.2
 The physician orders Mezlin (mezlocillin) 50 mg/kg every 4 hours IV for infection. The child weighs 60 lb. The pharmacy sends a vial of medication labeled Mezlin 1 g. The nursing drug reference provides information to reconstitute 1 g of medication with 10 mL of sterile water for injection, 0.9% NaCl, or D5W.
The physician orders Mezlin (mezlocillin) 50 mg/kg every 4 hours IV for infection. The child weighs 60 lb. The pharmacy sends a vial of medication labeled Mezlin 1 g. The nursing drug reference provides information to reconstitute 1 g of medication with 10 mL of sterile water for injection, 0.9% NaCl, or D5W.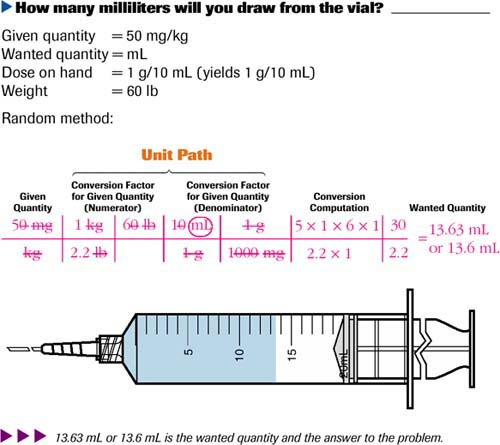 |
Exercise 5.2 Medication Problems Involving Reconstitution (See pages 137–138 for answers)
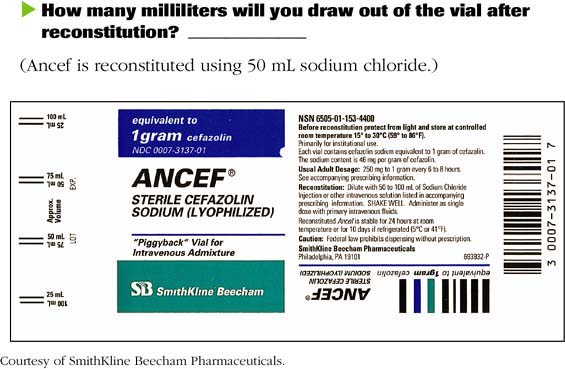
Order: Ancef 500 mg IV every 8 hours for infection.
Order: Primaxin 250 mg IV every 6 hours for infection
Supply: Primaxin vial labeled 500 mg. Reconstitute with 10 mL of compatible diluent and shake well.
How many milliliters will you draw from the vial after reconstitution? _____________
 Order: Unasyn (ampicillin) 50 mg/kg IV every 4 hours for infection
Order: Unasyn (ampicillin) 50 mg/kg IV every 4 hours for infection
Supply: Unasyn 1.5-g vial
Nursing drug reference: Reconstitute each Unasyn 1.5-g vial with 4 mL of sterile water to yield 375 mg/mL.
The child weighs 40 kg.
How many milliliters will you draw from the vial after reconstitution? _____________
Order: erythromycin 750 mg IV every 6 hours for infection
Supply: erythromycin 1-g vial labeled: Reconstitute with 20 mL of sterile water for injection.
How many milliliters will you draw from the vial after reconstitution? _____________
 Order: Fortaz 30 mg/kg IV every 8 hours for infection
Order: Fortaz 30 mg/kg IV every 8 hours for infection
Supply: Fortaz 500-mg vial labeled: Reconstitute with 5 mL of sterile water for injection
The child weighs 65 lb.
How many milliliters will you draw from the vial after reconstitution? _____________
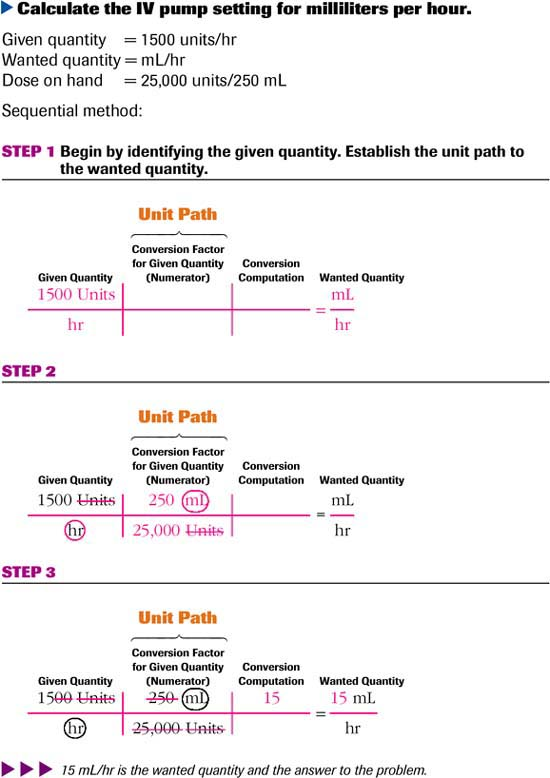
Medication Problems Involving Intravenous Pumps
IV medications are administered by drawing a specific amount of medication from a vial or ampule and inserting that medication into an existing IV line. All IV medications must be given with specific thought to exactly how much time it should take to administer the medication. Information regarding time may be obtained from a nursing drug reference, label, or package insert, or may be specifically ordered by the physician.
Although IV medications can be administered IV push, the time involved often requires the use of an IV pump. All IV pumps deliver milliliters per hour (mL/hr) but may vary in operational capacity or size.
Below is an example of the dimensional analysis problem-solving method with basic terms applied to a medication problem involving an IV pump.
 Preventing Medication Errors
Preventing Medication ErrorsWhen administering IV medications and fluids, always check a nursing drug reference to obtain the correct information regarding how much medication or fluid can safely be administered to prevent medication errors.
It is the responsibility of the nurse to be familiar with the different types of IV pumps that are used to deliver IV medications or fluids. All medications delivered by the IV route should be delivered using an IV pump to ensure accuracy and safety of delivery.
 |
 Thinking it Through
Thinking it ThroughStay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree




 Order: Claforan 50 mg/kg IV every 8 hours for infection. The infant weighs 12 kg.
Order: Claforan 50 mg/kg IV every 8 hours for infection. The infant weighs 12 kg.